MODULE: Monge's Theorem.

Part 4: Dilations.

We would like to present a different view of the construction of Monge's Theorem. This involves the use of "dilations", that is, transformations of the plane that are either contractions or expansions about a point (the "center of the dilation"), by a constant (positive) ratio. A dilation can either be an expansion (if the ratio is larger than one) or a contraction (if it is smaller than one).

Construct a point C in the plane, and mark it as the center of dilation. Now draw any polygonal figure, and dilate it about the center C by a fixed ratio (1/2, or 3, or whatever). Drag around this polygon, and observe how the image changes. In particular look at the vertices, their images and the center. Can you see any relation among them?

We look now at the converse problem. That is, if we are given a figure and its image under a dilation, can we find the dilation?

Suppose you have a point A, and its image A' under a certain (unknown) dilation. Where would the center of the dilation have to be? Is there only one possibility? Construct two points, and then find several dilations (that is, combinations of centers and ratios) giving the second point as image of the first one. (It is convenient here to use dilations by a "marked ratio". Once we pick the center, what would this ratio be?)

What if now we have two points A and B and their images? Assuming the points and the images are not all on one line, how can you find the center of dilation? What should you use as a ratio? We saw that just one image does not completely determine the dilation. Can you give the two images arbitrarily, and still get a dilation?

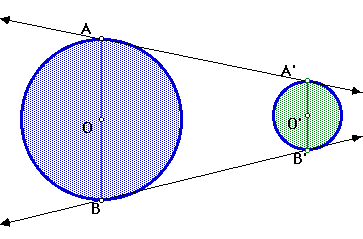
Returning to Monge's theorem, start now with two circles of different radii. Is there a dilation so that the image of one of the circles is the other one? Where is the center? Can you construct it? Does the figure look familiar? Check that the dilation really maps one circle onto the other. How is the center of dilation related to the center of symmetry discussed in Section 2?

Now let's look at what happens when we "compose" two dilations, i.e., what happens if we follow a dilation by another one.

Draw two points, C1 and C2, to be used as centers. Draw a figure to dilate. Mark C1 as center, and dilate the figure by some fixed ratio. Now mark C2 as center, and dilate the figure resulting from the first dilation about this center, by some other ratio. (Just be careful only that the product of the two ratios is not 1). Move the original figure around, and see how the final image changes. Again, it is convenient to restrict your attention to only a pair of vertices. What relation does the final image seem to have with the original figure? If it is a dilation, how would you find the center? Do you see any relation between the two original centers and this new one?

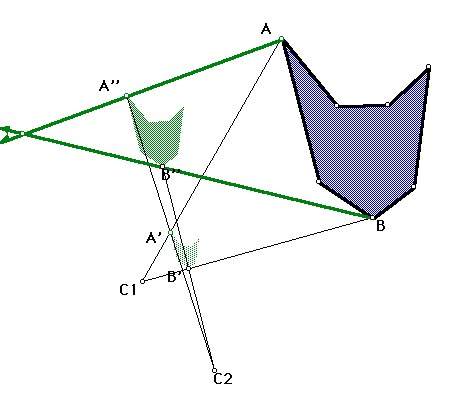
Repeat the above construction, but now with the product of the ratios equal to 1. What happens now with the final figure? Where is the "new center"? Can you guess the relation between the original and the final image? Check whether you are right or not.

Let us return to the case when the product of the ratios is not one, and consider the net effect of doing a pair of dilations. This is another dilation, called the composition of the first two. What can we say about the location of its center (the "new center")? As before, construct two points C1 and C2 to be used as centers of dilations. Now construct the line between them, and a point P on it. Look at what happens to P under the two successive dilations. Where is the first image going to be? What about the final one? Considering the original point P and its final image, where must the center of the composition be?

These last observations have in them all the ingredients necessary for showing why Monge's theorem is true.

Consider now the set-up of Monge's Theorem. Given three circles of different radii, we wish to show that the centers of symmetry of the three pairs of circles are collinear. We can do this by reinterpreting the problem in terms dilations.

Consider the dilation that takes the first circle to the second. If we compose it with the dilation taking the second circle to the third, we get a dilation that takes the first circle to the third. Use what you have learned about centers of symmetry, dilations of circles, and composition of dilations to prove Monge's Theorem.

The Geometry Center Home Page

Author: Eduardo Tabacman, revised and edited by Evelyn Sander
Comments to: webmaster@geom.umn.edu
Created: Jun 09 1996 --- Last modified: Jun 11 1996