Triangle Sum Conjecture
Activity Sheet
The objectives for this activity sheet are:
- To determine your understanding of the Triangle Sum Conjecture. Typical geometric problems requiring the ideas of this conjecture, coupled with ideas from previous conjectures, are given for you to solve.
- To give you the opportunity to explore this conjecture further through construction activities involving:
- Geometer's Sketch Pad
- Compass and Straight Edge
Solving Geometric Problems
Use the Triangle Sum Conjecture to find the missing values in the diagram below.
(Watch out! You may need the ideas from previous conjectures as well!!)
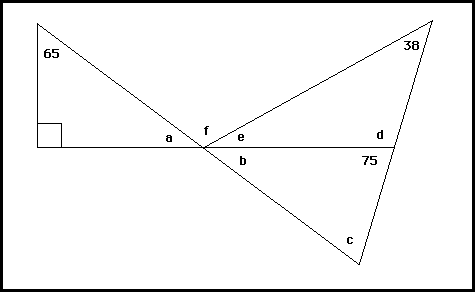
- a = ??
- b = ??
- c = ??
- d = ??
- e = ??
- f = ??
Click here for the solutions.
Further Investigations with Geometer's Sketch Pad
Directions: Open the Geometer's Sketch Pad program on your computer. Then follow these directions:
- Construct a triangle ABC with three line segments: AB, BC, and CA.
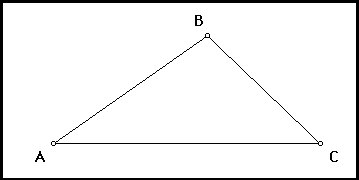
(Your sketch should look like this.)
- Measure each angle using the Measure Menu. Record each of these values.
- Angle ABC = ???
- Angle BCA = ???
- Angle CAB = ???
- Add the measures of these three angles by selecting each of them, and using the Measure/Calculate Menu. What is the sum of their measures?
- Use the mouse to drag any of the vertices of the triangle. Note what happens to the sum of the angle measurements.
- What happens?
Are you convinced?
Compass and Straight Edge Activity
- Use a straight edge to construct a triangle ABC so that it looks like the picture above.
- Draw a horizontal ray OP on the bottom of your paper. The ray should start near the center. (See the pink ray OP in the diagram below)
- Copy (with straight edge and compass) the angle CAB so that the ray OP is one of the sides of the copied angle. (see the new blue ray in the diagram below)
- Next copy angle ABC so that the newly constructed ray is one of the sides of the copied angle. (See the new green ray in the diagram below)
Note: You have just added CAB + ABC
- Finally, copy the angle BCA so that the newest constructed ray is one of the sides of the copied angle. (See the black ray in the diagram below)
Note: You have added the three angles, CAB + ABC + BCA
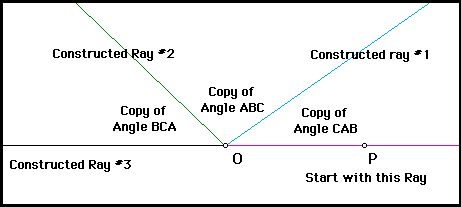
(Your construction should look like this)
- Using a protractor, measure the angle the angle between the first (pink) and last (black) constructed rays. Is it 180?
- If you did not get a total of 180, what do you think happened?
- Try constructing another triangle, perhaps one with an obtuse angle, and repeat the angle copying constructions given above.
- What happens? Do you get 180?
Are you convinced?
Back: Conjectures in Geometry home page or to the List of Conjectures.
Back: Explanation of the
Triangle Sum Conjecture.
Next Conjecture: Explanation of the Quadrilateral Sum Conjecture
Next Activity: Activities for Quadrilateral Sum Conjecture
Solutions to Problems
- a = 25
- b = 25
- c = 80
- d = 105
- e = 37
- f = 118
Back to the Problem Set