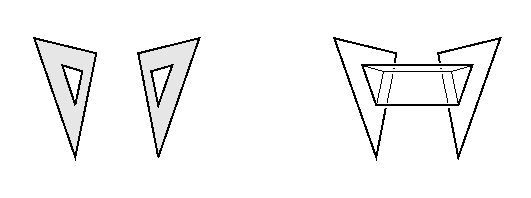
Given a tight surface, it is always possible to add a handle to the surface without losing tightness. For the polyhedral case, we can describe this process in more detail. Take any two faces that are not co-planar, remove a triangle from the center of each (below, left), then add a triangular tube between these two holes (right).
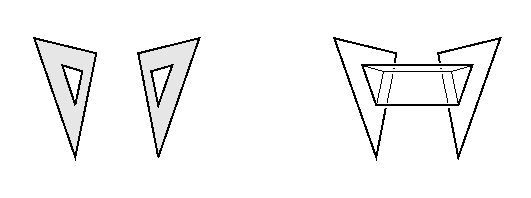
The resulting surface is still immersed (since the stars of the new
vertices are embedded
![[More]](pix/Link.gif) )
and tight (since the new vertices are not
extreme vertices
and the new handle does not change the convex envelope of the surface
)
and tight (since the new vertices are not
extreme vertices
and the new handle does not change the convex envelope of the surface
![[More]](pix/Link.gif) ).
).
Depending on the orientations of the initial triangles, the added handle can be orientation preserving, or orientation reversing, as in the diagram below:
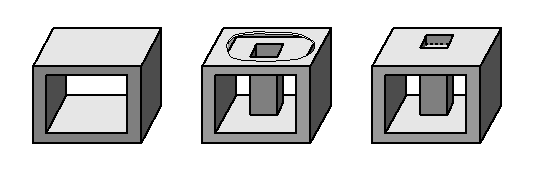
This picture shows a tight torus (left), with an orientable handle added (middle) or a non-orientable handle (right). The middle surface is a torus of genus 2 (a 2-handled torus), but the right-hand surface is non-orientable.
To determine what surface it is, we use the Euler characteristic. Note that the Euler characteristic must be the same for the middle and right-hand surfaces, since they have the same number of faces, edges and vertices). Since a 2-handled torus has Euler characteristic -2, so must the non-orientable surface at the right. Since this is even, it must be based on the Klein bottle, and in fact it is a Klein bottle with a handle. Kuiper gave a smooth version of this surface in his paper:
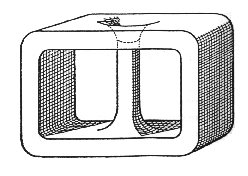
This represents a tight immersion of the Klein bottle with one handle. More handles can be added in the same way to obtain tight immersions of all the surfaces based on the Klein bottle. Other than the Klein bottle itself, this takes care of all the non-orientable surfaces with even Euler characteristic.
To do the same for the surfaces with odd Euler characteristic, Kuiper
needed a tight immersion of the real projective plane possibly with some
handles, and the fewer handles, the better. He was able to describe a
smooth projective plane with two handles
![[More]](pix/Link.gif) to which more handles could be added as above to obtain tight immersions of
all surfaces with odd Euler characteristic less than or equal to -3.
to which more handles could be added as above to obtain tight immersions of
all surfaces with odd Euler characteristic less than or equal to -3.
Kuiper does not give an explicit parametrization of his projective plane with two handles, so it is not very easy to visualize. Wolfgang Kühnel and Ulrich Pinkall [KP1] give an explicit description of a polyhedral version of the real projective plane with two handles. Their model has three-fold rotational symmetry, and they give a method of adding handles to this surface that maintains the symmetry. In addition, they provide a smoothing algorithm that can be used to generate smooth surfaces from certain polyhedral ones in such a way that tightness is preserved. Thus their polyhedral model can be used as the basis for a tight smooth surface with the same symmetries.
This leaves only three surfaces yet to consider: the Klein bottle with no handles (Euler characteristic 0), the real projective plane with no handles (Euler characteristic 1), and the projective plane with one handle (Euler characteristic -1).
![[Right]](pix/Right.gif) There is no tight projective plane
There is no tight projective plane
![[Right]](pix/Right.gif) There is no tight Klein bottle
There is no tight Klein bottle
![[Left]](pix/Left.gif) Orientable tight surfaces
Orientable tight surfaces
![[Up]](pix/Up.gif) Kuiper's initial question
Kuiper's initial question
7/20/94 dpvc@geom.umn.edu --
The Geometry Center