![[More]](pix/Link.gif)
![[More]](pix/Link.gif)
The boundary curves mentioned in (3) are called
top cycles, and they
play a key role in understanding tight immersions. Cecil and Ryan
[CR1] showed that the number
of top cycles of a tight immersion (of a connected surface other than
a sphere) is always at least 2 and no more than 2 - X(M),
where X(M) is the
Euler characteristic
of the surface.
![[More]](pix/Link.gif)
In the case of the Klein bottle, which has Euler characteristic 0, there must be exactly two top cycles, which we can assume lie in parallel planes. The M+ region is a sphere minus two disks, so is topologically a cylinder. The M- region joins the two top cycles, and since the Klein bottle is non-orientable, it must do so in a way that reverses the orientation relative to the outer cylinder.
Suppose such an M- region exists, and consider the slices of this surface by planes parallel to the two top cycles. As we move the plane from one top cycle to the other, the intersections start as a circle oriented one direction and must end as a circle oriented the other direction. Such a sequence would represent a smooth homotopy of a circle to a reversed circle, which is known not to exist. This is a contradiction, so there is not tight immersion of the Klein bottle.
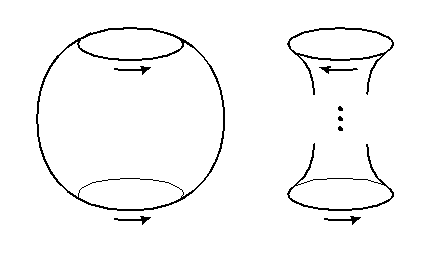
(This is not actually quite true, and this is not really what Kuiper
did. If the sequence above passes a saddle point, the number of
components in the slice changes, and the result is no longer a
homotopy. Since almost all height functions in a tight immersion are
polar
![[More]](pix/Link.gif) ,
almost every direction will have exactly two saddle points, and in
particular, this will be true for some direction nearly perpendicular
to the plane of the top cycles. What Kuiper actually does is show
that, under these conditions, the orientation of the circle at one top
cycle must be the same as that at the other, by indicating how the
orientation can be preserved at each saddle point.)
,
almost every direction will have exactly two saddle points, and in
particular, this will be true for some direction nearly perpendicular
to the plane of the top cycles. What Kuiper actually does is show
that, under these conditions, the orientation of the circle at one top
cycle must be the same as that at the other, by indicating how the
orientation can be preserved at each saddle point.)
Kuiper's original proof [K2] was for smooth surfaces, but he later extended this to polyhedral surfaces as well [K3].
![[Right]](pix/Right.gif) There is no tight projective plane
There is no tight projective plane
![[Left]](pix/Left.gif) Non-orientable tight surfaces
Non-orientable tight surfaces
![[Up]](pix/Up.gif) Kuiper's initial question
Kuiper's initial question
7/21/94 dpvc@geom.umn.edu --
The Geometry Center