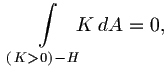
![[LINK]](pix/Link.gif) .
In showing that the total absolute curvature is bounded below
by 4 - X(M)
.
In showing that the total absolute curvature is bounded below
by 4 - X(M)
![[More]](pix/Link.gif) ,
where X(M) is the
Euler characteristic
of the surface,
the inequality is introduced when an integral is divided into two
non-negative parts, one of which is simply dropped. To have minimal
total absolute curvature, this
dropped integral must actually equal zero; so a surface is tight if,
and only if,
,
where X(M) is the
Euler characteristic
of the surface,
the inequality is introduced when an integral is divided into two
non-negative parts, one of which is simply dropped. To have minimal
total absolute curvature, this
dropped integral must actually equal zero; so a surface is tight if,
and only if,
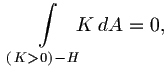
Since the integrand is always positive, the only way this integral can be zero is for the region of integration to be empty. This means that all the positive curvature must be on the convex envelope of the surface, an observation that leads to the following important geometric description of tight surfaces:
Theorem: An immersion of a closed, compact, connected surface M is tight if, and only if, it can be decomposed into two components, M+ and M-, such that
For example, the torus of revolution is tight (below left). Its decomposition into the M+ and M- regions is shown (right). Notice the two top cycles that form the common boundary of the M+ and M- regions.
![[Right]](pix/Right.gif) Top sets and top cycles
Top sets and top cycles
![[Right]](pix/Right.gif) Tightness and polar height functions
Tightness and polar height functions
![[Left]](pix/Left.gif) Tightness and its consequences
Tightness and its consequences
![[Up]](pix/Up.gif) Kuiper's original question
Kuiper's original question
8/8/94 dpvc@geom.umn.edu --
The Geometry Center