KALEIDOTILE FAMILY SOLIDS TABLE

(2,3,3) SYMMETRY GROUP

F = # of faces E = # of edges V = # of vertices

Model

F

E

V

Shapes

Tetrahedron
	4	6	4	4
Truncated Tetrahedron
	8	18	12	4 4
Octahedron (Tetratetrahedron)*
	8	12	6	4 4
Truncated Tetrahedron
	8	18	12	4 4
Tetrahedron
	4	6	4	4
Cuboctahedron (Rhombitetratetrahedron)*
	14	24	12	4 4 6
Truncated Octahedron (Rhombitruncated tetratetrahedron)*
	14	36	24	4 4 6

*The names in the parenthesis will be explore further in Lesson 12: Naming Polyhedra

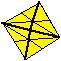
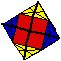
We have picked tetrahedron and rhombitetratetrahedron to show the 2-, 3-, and 3- fold symmetries in this group. In each of the polyhedra below, we have highlighted some planes of reflection symmetry to show the folds of symmetry.

2-fold	3-fold	a different 3-fold