For linear motion, Newton's second law relates the acceleration of a particle of mass m to the force F applied to it. In symbols,
F = m a .
From the equation (and common sense) it is clear that a larger force is required to accelerate a more massive particle. Intuitively, the mass of the particle resists acceleration. We say that the mass gives the particle linear inertia.
For angular (rotational) motion, the angular acceleration depends not only on the applied force, but also on where the force is applied in relation to the axis of rotation (or "pivot point"). For this reason, as we observed in the last section, it is the torque a force generates which is most naturally related to the angular acceleration of the particle.
Torque plays the same role in rotating systems that force plays in linear motion. However, the resistance to angular acceleration again depends on both the mass of the particle and its distance from the axis of rotation. Therefore we introduce a new quantity called the moment of inertia to measure resistance to angular acceleration. The moment of inertia of a rotating system is analogous to the mass of a linearly accelerating system.
Using Newton's second law, one can show that the relationship between the torque, T , and the angular acceleration, A , of a particle is given by
T = (m r 2) A .
Thus, we define the moment of inertia to be the quantity mr 2 of the particle. The moment of inertia is typically denoted by I .
In general, computing the moment of inertia can be quite difficult, requiring the more sophisticated techniques of iterated integrals from multivariable calculus. However, when an object is sufficiently symmetrical, we can compute the moment of inertia with a standard one-variable integral.
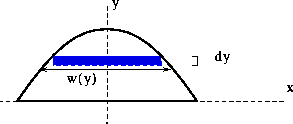
Now consider a planar object as in Figure 4, and imagine rotating it about the x -axis. (Note it is rotating out of the xy -plane into the third dimension perpendicular to your computer screen.) This might represent, for example, a model of a rotating turbine blade as it rotates around its central spindle.

In this case, note that the entire strip indicated in the figure remains at a constant distance from the axis of rotation. Therefore, this strip has the same moment of inertia as a particle of the same mass lying at the same distance above the x -axis.
If we assume that the object has been cut out of some thin material of uniform density p , the mass of the strip is simply p w(y) dy . Consequently, it's moment of inertia is
p y 2 w(y) dy .
It follows that the moment of inertia for the whole object is given by the formula

where a and b are the minimum and maximum values of the y variable.
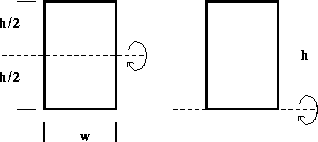
Answer the following questions by interacting with the Exploring Moments of Inertia page.
Copyright 1996 by The Geometry Center. Last modified: Fri Apr 12 15:53:58 1996