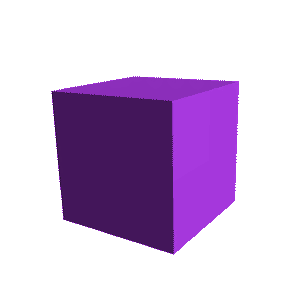
6 square faces
8 vertices
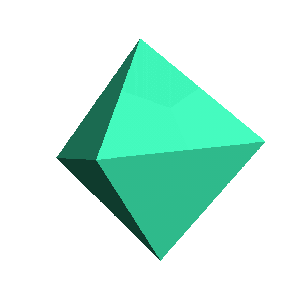
8 triangular faces
6 vertices
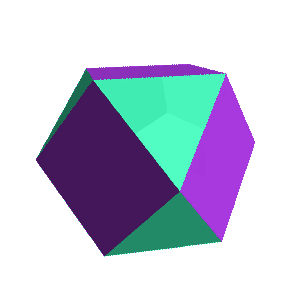
8 triangular faces
6 square faces

6 square faces 8 vertices |

8 triangular faces 6 vertices |

8 triangular faces 6 square faces |
|
If, when truncating the vertices of either the cube or the octahedron, we move in exactly half way, the result is the cuboctahedron. Thus, we can think of the cuboctahedron as being the limiting case of either the truncated cube or the truncated octahedron.
This happens because the cube and octahedron are dual solids. Notice that the cube has 6 faces and 8 vertices, while the octahedron has 8 faces and 6 vertices. Other Platonic solids which are duals are the icosahedron and the dodecahedron--see the icosidodecahedron page. The tetrahedron is self-dual; it has 4 vertices and 4 faces.