0.01 cos^2(t) - 0.01 sin^2(t) + 1.This function oscillates between 0.99 and 1.01, so sometimes it is greater than f(0,0) and sometimes it is less. This is indicative of the neighborhood of a saddle point: for a saddle point there will be two "sectors" of direction in which the function is less than the critical value, and two sectors of directions in which the function is greater than the critical value.
If the critical point were a minimum, then we would expect the restriction of f to a small circle about the critical point to always be greater than the critical value.
b) No critical points.
c) Critical point at (1,2). D=-5. Saddle
d) Critical point at (0,0). D=0, so the test fails. Evaluating the function on a small circle shows that the critical point is a minimum.
e)There are two critical points. The one at (0,0) is a saddle. The one at (2/3,-2/3) is a local maximum.
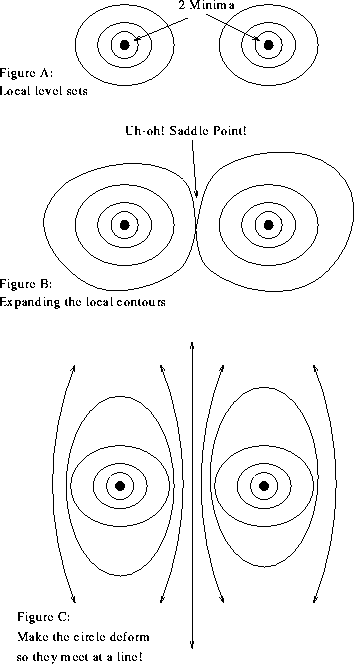
One correct answer is that a function with exactly two isolated critical points, both minima, does exist! A sample answer is below (a good "partially correct" answer stops at Figure B). Notice, however, that the example function has an entire line of critical points, so it is also correct to draw Figure 3 and say "no, because there is a whole line of critical points.
If you actually tried to write down a formula for such a function, you probably didn't get far. Formulas do exist, but you need to construct them by understanding the geometry of surfaces.