An Investigation of
the Externally Tangent Circles to a Triangle
Background
In this section of the exploration you will be asked to discover the way you can construct externally tangent circles based on the properties of the angle bisector. As you work through the material, you may be asked to write down the answers to the questions in the investigation or to save the information and your sketch on a disk. Ask your teacher for specific directions.
Exploring the Properties of Externally Tangent Circles to a Triangle
A circle is externally tangent to a triangle if it touches one of the sides of the triangle and the extensions of the other two sides.
Investigation
Let us open the sketch with triangle ABC, with extended sides AB and AC. Click here to activate a sketch. After the sketch appears follow the instructions.
- Construct the angle bisector of angle PBC.
- Construct the angle bisector of angle QCB.
- Construct the intersection point D of the two bisectors. Rename it if necessary. Construct the perpendicular segments from D to the ray AP,from D to the ray AQ, and from D to the segment BC. What do you expect to be the relationship between their lengths? Why?
- Measure the lengths of the perpendicular segments. What do you observe? Is the answer what you have expected? Move the vertices of the triangle and compare again the measures of the perpendicular segments.
- Construct the circle with center -- point D and radius -- the length of the perpendicular segment from D to BC. How would you call this circle? Move the vertices of triangle ABC. What do you observe is happening to the circle?
- Click here to check your answers.
The next step in our construction is to draw all externally tangent circles to a triangle. You may use this script to construct the circles externally tangent to this triangle .
Click here to compare your drawing.
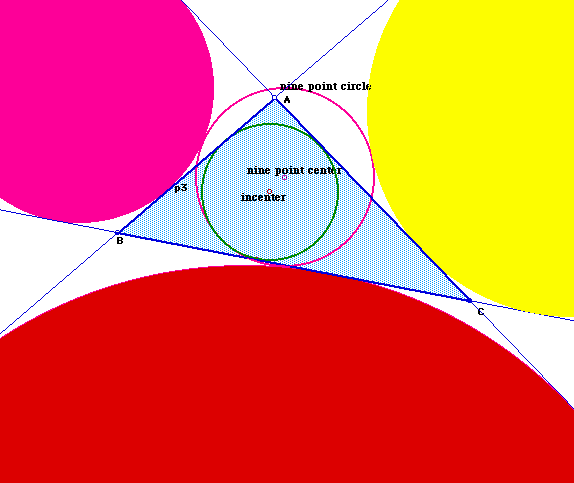
Before we finish this section let us consider an application of the externally tangent circles. Have you heard of the nine point circle? If you have not, you should go back to the main menu and investigate the amazing properties of this famous object. Look at the picture below. What you see is yet anothet property of the nine point circle! What is it? Make a conjecture. Click here to check your answer.
Before you leave this investigation....
Did you follow your teacher's directions about saving your work and turning it in?
Next: Go Back