
The Archimedean method for calculating  made
use of the same idea as did
the Babylonian method. Archimedes, however, used the concept of making
successive approximations and ascertained that the number
made
use of the same idea as did
the Babylonian method. Archimedes, however, used the concept of making
successive approximations and ascertained that the number  could be determined, in principle, to a
ny degree of accuracy desired. He took a circle and inscribed a regular
hexagon within the circle and circumscribed one about the circle. He
then, by using successive bisections, inscribed and circumscribed
regular polygons within and about the circle with sides of 12, 24, 48,
and finally 96 sides. The regular polygons circumscribed about the
circle formed convergent upper bounds for the circumference while the
regular inscribed polygons formed convergent lower bounds. The third
century B.C. value which Archimedes obtained without decimal or
positional notation and without trigonometry attests to his tenacity.
(3 10/71 <
could be determined, in principle, to a
ny degree of accuracy desired. He took a circle and inscribed a regular
hexagon within the circle and circumscribed one about the circle. He
then, by using successive bisections, inscribed and circumscribed
regular polygons within and about the circle with sides of 12, 24, 48,
and finally 96 sides. The regular polygons circumscribed about the
circle formed convergent upper bounds for the circumference while the
regular inscribed polygons formed convergent lower bounds. The third
century B.C. value which Archimedes obtained without decimal or
positional notation and without trigonometry attests to his tenacity.
(3 10/71 <  < 3 1/7) [2].
< 3 1/7) [2].
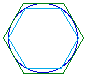
To see how an inscribed hexagon and a circumscribed hexagon gives lower
and upper bounds for  click on the Geometer's
Sketchpad inscibed and circumscribed hexagons sketch.
click on the Geometer's
Sketchpad inscibed and circumscribed hexagons sketch.
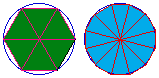
To see how approximations of  improve as the
inscibed polygon
doubles in the number of sides click on the Geometer's Sketchpad hexagon vs dodecagon sketch.
improve as the
inscibed polygon
doubles in the number of sides click on the Geometer's Sketchpad hexagon vs dodecagon sketch.
These two ideas - inscribed and circumscribed polygons and increasing
the number of sides of the polygons - are put together into the Archimedean method sketch. In this sketch
you can change the angle that gives a (360°/angle)-sided polygon.
(Realize that the method used allows for non-integral number of sides.)
By increasing the number of sides, what happens to the lower and upper
bounds for  ?
?
Return to Historical Overview of Pi

http://www.geom.umn.edu/~huberty/math5337/groupe/archimedean.html Copyright © 1996-1997 Michael D. Huberty, Ko Hayashi & Chia Vang
Created: March 1996 ---- Last Modified: July 6, 1997